A typical position-control hydraulic servo consists of a control valve (either a servovalve or a high-response proportional valve), hydraulic cylinder with a position-feedback transducer, and an electronic controller to close the control loop. Loop gain is the product of all component gains in the loop when the feedback path is open. The dynamics in the loop act on the open-loop gain as the input signal frequency increases. These dynamics cause the loop gain to change with signal frequency, and they introduce phase lags into the loop.
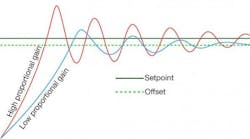
The allowable loop gain is the maximum loop gain that produces a control-loop dynamic behavior to meet specified stability criteria so that a stable output response results when the loop is closed. Because loop gain is sensitive to signal frequency, it typically is specified as the loop gain at a reference frequency of 1.0 rad/sec. The allowable loop gain will determine the servo’s static and dynamic position-control accuracy. Therefore, the allowable loop gain should be as high as possible.
Investigating resonances
The dynamics of interest in this situation — those influencing position-control loop dynamic behavior — can be reduced to an integration and a dominant resonance. The integration is inherent in position-control loops because the open-loop cylinder position is determined by the time integral of cylinder velocity. A dominant resonance is the resonance with the lowest natural frequency of all resonances in the loop and is separated in natural frequency from the natural frequencies of the other resonances by a factor of 2.5 or greater. With this degree of natural-frequency separation, the dynamics of the other resonances will have only minimal influence on control-loop dynamic behavior, so they can be neglected.
The cylinder and the control valve account for the significant resonances in the control loop. Establishing one of these resonances as the dominant resonance greatly simplifies the control-loop dynamic analysis and will ultimately result in the highest allowable loop gain. Therefore, once the cylinder and control valve are selected and sized to meet the load-driving requirements, the cylinder’s natural frequency should be calculated (using equations from hydraulics references).
The natural frequency (90° phase lag frequency) of the selected control valve must be adequate to establish either the cylinder resonance or the control valve resonance as the dominant resonance. Due to driven-mass loads reflected to the cylinder, cylinder natural frequencies will generally be 15 Hz or less, whereas servovalve or high-response proportional valve natural frequencies are much greater, so cylinder resonances generally dominate. (An exception may be when the mass-load reflected to the cylinder is small. In this case, the cylinder’s natural frequency can be very high and the control valve resonance will be dominant.)
When the lightly damped cylinder resonance is dominant, linear-control theory can be used to show that gain margin stability criteria apply and that the allowable loop gain can be calculated from the equation:
Kg = 6.28 × ζc × We
Where Kg is the allowable loop gain, sec-1
ζc is the cylinder’s damping ratio (less than 0.50), and
Wc is the cylinder’s natural frequency in Hz.
When the heavily damped control-valve resonance is dominant, linear-control theory can be used to show that phase margin stability criteria applies, and the allowable loop gain can be calculated from the equation:
Kg = 2.39 × Wv
Where Wv is the control valve natural frequency in Hz.
The allowable loop gain for position-control hydraulic servos can vary widely, depending upon the specific application requirements. However, our experience indicates that a typical allowable loop-gain range for mobile hydraulics is 7 to 15 sec-1and 15 to 30 sec-1 for general industrial applications.
Note that allowable loop gains from the two equations are proportional gains. There will be some applications where proportional gain only is not high enough to meet the control-accuracy requirements. In these cases, some means of electronic gain compensation can be included in the electronic controller. Allowable loop gain increases by factors from 3 to 10 are not uncommon. When such a situation occurs, the electronic controller supplier should be consulted to provide the necessary gain compensation capability.