Every pneumatic device possesses a specific conductance, identified as Cv . Because Cv is perceived as a complex expression, many designers avoid learning about it and, hence, become ignorant of its importance and proper application. Pneumatic conductance expresses the ability of a fluid to flow under a pressure differential ( ••• and is often referred to as flow capability or, more commonly, as the flow coefficient. Conductance is inversely proportional to resistance and is, in fact, its reciprocal. Put simply, Cv expresses the flow capability of any fixed-orifice pneumatic device, such as a valve, cylinder port, or fitting. Conductance values in pneumatics, as in electricity, can be combined to render system conductance, Cvs .
Electrical and pneumatic conductance
As with pneumatics, every electrical device in a room has a resistance — and its reciprocal, conductance, G . Conductance can be calculated for each device and combined to determine total conductance for the room. The individual and combined current draw can then be calculated based on conductance and the impressed voltage in the room's circuit. This information allows sizing branch and trunk lines to feed all appliances. You can then ascertain the size of the main switch and the specific switches for each appliance or outlet in the room. Doing the same for other rooms yields a total for a household or business. Depending on the safety factor involved, these calculations can be repeated for all homes and businesses to determine generating capacity required for an entire city.
The same procedure should be followed for pneumatic systems for the very same reason: namely, to determine loads and requirements for sizing components. Combining s of every pneumatic component reveals total conductance for a machine. As with electricity, this allows determining flow requirements based on conductance and available pressure. This, in turn, allows selecting the main conductor and individual lines. Next, we can calculate the size of the main valve and all subordinate valves. (A pneumatic valve is the equivalent of an electrical switch, which, in this case, either allows or prevents air from passing.) Finally, we can calculate the needs of other machines to determine a facility's required compressor capacity — the pneumatic equivalent of an electric generator.
Expressing all fixed-orifice pneumatic devices in terms of their conductances is like having all monetary systems designated in so many ounces of gold so that dollars, francs, lire, pounds, and other currencies can be compared to one another for evaluation and exchange. Analogous to this, conductance allows comparing the flow capability of one device to another for sizing, selection, and rating.
The conductance of a device plays the same general role in pneumatics as it does in electricity. Nowhere is it as clearly demonstrated as in the comparison of the following two basic equations. The first equation, for electricity, is Ohm's familiar law; the second is the basic flow equation at critical flow for pneumatics:
E = I x R ,
where E is impressed voltage in volts,
I is electrical current in amperes, and
R is electrical resistance in ohms.
Rearranging Ohm's law produces:
I = E / R .
But, again, conductance is the reciprocal of resistance; so, substituting:
I = G E .
Applying this electrical analogy to pneumatics yields:
Q = 0.489 x p1a ,
where Q is air flow in scfm,
0.489 is a constant for air at standard conditions (14.7 psia, 68° F, 36% relative humidity),
Cv is conductance of any fixed-orifice pneumatic device, and
p1a is the maximum upstream pressure available to the device in psia.
Examining these two basic equations reveals a striking similarity. The same relationship exists between transmitting electrical signals and pneumatic signals through their respective conductors. In the context of electricity, the term impressed voltage implies pressure, and, thus, its counterpart in the flow equation is pressure. Conductance carries the same meaning in each discipline, so Cv is substituted for G . Finally, I , representing current (the flow of electrons expressed as amperes), has Q as its parallel, which denotes flow of air molecules expressed in scfm.
Although no universal attempt has been made in pneumatics to assign a term equivalent to resistance, its reciprocal, conductance, is expressed as a dimensionless number simply known as Cv. Do not confuse this pneumatic Cv with the thermodynamic cv , which refers to specific heat of a fluid at constant volume.
Finding system conductance
Comparing the mathematics for determining the combined conductance and resistance as they relate to pneumatics and electricity further indicates the close affinity between these two subjects. Calculating system conductance, Cvs, for devices connected in parallel is accomplished simply be adding together the conductances of each individual component. However, to calculate conductance of pneumatic devices connected in series, the following equation applies:
Cvs =1 / (1 / Cv12 + 1 / Cv22 + . . . 1 / Cvn2)1/2
To combine pneumatic resistances in series, the following equation applies:
Rs = (Rp12 + Rp22 + . . . Rpn2)1/2
For example, assume that three pneumatic components, with Cvs of 1, 2, and 3, are arranged in series. Therefore, their individual resistances (reciprocals) are 1, l/2, and l/3, respectively. Their combined conductance is:
1 / (1/12 + 1/22 + 1/32)1/2 = 0.857 Cvs.
The reciprocal, resistance, is 1.17
As a check, we also calculate pneumatic resistance:
[(12) + (2)2 (3)2]1/2 = 1.17
Its reciprocal, conductance, is 0.857.
It should be evident that adding any device to an existing series circuit reduces the overall flow coefficient, because doing so increases the total resistance. Further, it follows that removing a device from a series circuit increases the system conductance, because doing so reduces the total resistance. The order in which devices are located in a series circuit has no effect on the final result.
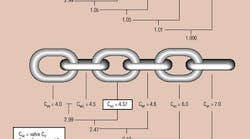
Finding the weak link
To gain further insight, compare a component's conductance to the strength of a single link of a chain and the system conductance to the strength of the entire strand of chain. This is not an exact analogy, but is convenient and easy to relate to. The chain's strength is dictated by its weakest link, which must support the maximum load applied to it. So, too, the component with lowest Cv dictates the system's Cvs . This system Cvs is always less than the lowest component Cv . This is evident from the example with three devices, whose Cvs were 1, 2, and 3. When we they were combined, the result was 0.857. If only 1 and 2 were combined, the result would have been 0.894. The equation that reflects this phenomenon is the addition of reciprocals and applies to electricity as well.
This phenomenon means that no single component in a circuit is more significant than its neighbor. As in a chain, each link is as important as the next, and each must carry its load; if not, the entire network collapses. To increase capacity of a chain, we must replace or strengthen the weakest link. As a corollary to this, to improve the speed of a cylinder, we must eliminate the lowest Cv in the circuit by replacing or modifying the limiting component.
To understand another example, refer to the figure at the bottom of the page, which illustrates the analogy by representing Cv s of components in a pneumatic system as links in a chain. If a cylinder's piston moves too slowly, a larger valve often is specified in an attempt to rectify the problem. However, if the goal is to cut the cylinder's extend time in half, it should be apparent that increasing the valve Cv (size) renders only a negligible improvement to the overall system Cvs . The reason should be obvious — namely, the valve is not the weakest link. In order to improve the performance of the system, the weak link must be replaced — which means replacing or modifying the component with the lowest Cv .
In this example, the offending link turns out to be a restrictive orifice; supplanting it with a larger orifice will achieve the desired cycle time. No volume changes took place in any of the components; the only modification to reduce cycle time was to increase Cvs . The lesson here is that we should always calculate and display the Cv of every component of a circuit in a survey. We then can quickly identify the lowest Cv and take action to increase it.
Relating conductance to flow
Having gained an appreciation of Cv , let us review its fundamental importance. Air can travel at the speed of sound — approximately 1120 ft/sec under standard conditions — in the earth's atmosphere. The labyrinthine path in an air valve presents restrictions that reduce the velocity. Air velocity is further impeded through straight and, especially, elbow fittings. Even in a long, straight run of a conductor — the only component that affords an opportunity to acquire substantial speed — internal wall friction reduces average air speed to a maximum of about 500 ft/sec. This friction is a function of the surface roughness of the conductor's ID and the constraint imposed by the ID itself.
To visualize the potential for compressed air to attain high speed, consider a single air molecule from the galaxy of molecules contained in air. Now compare this to a car maneuvering through a race course. The car attains maximum speed on a straightaway, simply because there are no obstructions from curves or other impediments.
After it maneuvers its way through the many bends, twists, turns, and restrictions of a pneumatic system, the compressed air eventually reaches the actuator, where work is ultimately performed. In a cylinder, a piston is considered moving very fast when compressed air moves it as slowly as 10 fps because the endofstroke impact requires effective shock-absorption to prevent premature rupture. However, 10 ft/sec is actually less than 7 mph, and most actuators travel at speeds considerably lower than this. Nevertheless, a procedure is essential to determine performance time in order to predict cycle time and, ultimately, the number of finished pieces emanating from a production line. Cv is an effective tool for making that computation.
Cycle time is predictable for each cylinder of a machine. If specifications for the cyclic rate of a machine are not met during the design stage, components and/or pressures can be altered until the desired results are attained, which is where Cv comes in.
| An opportunity to reduce compressed air energy costs According to the DOE, compressed air systems account for 10% of all electricity and roughly 16% of U.S. industrial motor system energy use. Approximately 70% of all manufacturing facilities in the United States use compressed air to drive a variety of equipment. More than half of all industrial plant air systems hold potential for substantial energy savings with relatively low investment costs. In small-to medium-sized industrial facilities, approximately 15%of compressed air system usage can be saved with simple paybacks of less than 2 years. In larger facilities, these savings could range from 30 to 60% of current system usage. In addition to energy benefits, optimized compressed air systems frequently offer corresponding improvements in system reliability, product quality, and overall productivity. The DOE's compressed air assessment project sought to answer a number of key questions concerning the demand and supply sides of the market for compressed air efficiency services. Participants in the assessment included 91 compressed air equipment distributors and 222 industrial end users with compressed air systems and several compressed air system consultants. Findings on the demand side noted that awareness of and concern for compressed air efficiency is very low. Although system reliability was identified as a primary objective in compressedair system management, 35% of those interviewed reported that they had experienced unscheduled shutdowns of their compressed air systems during the previous 12 months. Sixty percent of these establishments had unscheduled shutdowns of two days or more. Fully 57% of manufacturing plants had taken no action to improve compressed air system efficiency in the two years prior to the survey. Supply-side findings revealed that more than three distributors out of four report that they offer system efficiency measures. While this is a growth area, efficiency services provide only an estimated 4% of their total revenue. Most distributors identified a general lack of understanding of the benefits of compressed air efficiency measures among customers. |