Last month’s edition of “Motion Control” showed how an unloading compensator is used with a fixed displacement pump to emulate a variable-displacement pump with load sensing — inefficiency notwithstanding. It also showed how pressure-reducing compensated flow controls in each section of a valve stack achieved independent flow control to each of several loads. These examples represent a useful hydraulic circuit design strategy. Perhaps more importantly, they create control systems that reduce the amount of operator training required to develop productive skills.
They are not, however, ideal systems. They are better than simpler circuits, but some characteristics may deviate considerably from the ideal. The aim this month is to explain the performance of the unloading compensator to reduce the number of surprises in an application and to help ensure that proper sizing and component selections can be made. There will also be some discussion on the math modeling of these valves, because all of the graphs have been generated using models, not actual test data.
Laying the groundwork
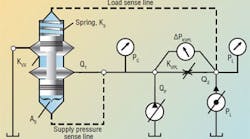
Figure 1 shows a combination analytical schematic, cutaway diagram, and ISO symbology for an unloader system that has been reduced to its most minimal form without sacrificing essential features. The figure will suffice for both an explanation of the normal laboratory test procedure as well as for development of a mathematical model.
Recall that the aim of the unloader design is to maintain a constant pressure drop across a metering orifice, KVPL, the powered land of the associated 4-way directional control valve. Thus, we see in Figure 1 that the Load sense line and the Supply pressure sense line connect to the two ends of the compensator spool such that the spool itself senses the differential pressure across KVPL.
It was argued that if the differential pressure drop was maintained constant across a given metering orifice opening, then the flow through it must be constant as well. Furthermore, if the metering orifice is variable, such as is the case of the 4-way spool, the amount of flow is adjustable by the operator merely by changing the amount of spool shift.
However, the pressure drop across the metering orifice is not constant. It varies by a considerable amount as the 4-way spool, load and supply pressures are varied. The result is that the flow is not constant for a given 4-way setting while load and supply pressures are changing. Actual testing reveals the degree to which the flow varies. Well-developed mathematical models will also predict the variability and provide insights into the causes.
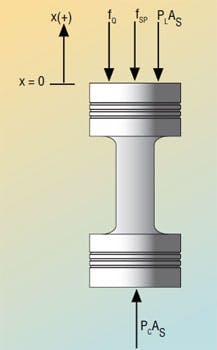
The free body diagram of Figure 2 is used to sum all the forces that significantly affect the movement and position of the compensator spool. In making models of things that are geometric, it’s necessary to establish a coordinate system that defines where zero is located. For the spool, fortunately, it is a one dimensional system, and only the x = 0 point needs to be set. It is located at the point where the compensator spool just begins to open, and it is positive in the opening direction.
The area that results from the opening of the compensator spool is commonly referred to as the “curtain area.” It is the flow through the compensator spool metering land that moves radially through a cylindrical geometry into an annular opening as it passes the compensator spool. That curtain flow area is approximately the circumferential area of the cylinder formed by the spool opening.
Effects of flow forces
In most valves — the instant one being no exception — flow force acts to close the valve. That may not be intuitive, but it is true, having been verified over the years with actual test data. In the unloader valve, this means that the flow force acts in the same direction as the bias spring. Therefore, some observers have stated that it acts as a non-linear spring that assists the bias spring. In other words, flow force tends to make the bias spring look stiffer. This is actually a good thing, but it also accounts for some of the less intuitive behavior of the valve in the application.
There is also the issue with the flow angle, which is the angle that the flow stream makes as it accelerates through the restriction offered by the partially opened compensator orifice. Some valve modelers use the simple, conventional interpretation that the flow angle is always at 69°, regardless of the degree of valve opening. In his classic text, Hydraulic Control Systems, published by John Wiley & Sons, Inc., Herbert E. Merritt states that the flow angle varies from 21° to 69° as the spool increases its opening from zero to maximum. Furthermore, the flow angle increases approximately in an exponential fashion. The rate of change is largely affected by the spool-to-bore clearance, which in, turn is a function of manufacturing tolerances. Therefore, the rate of change varies from valve specimen to valve specimen. Flow force, then, is somewhat of a random, unknowable quantity except when the clearances have specifically been measured.
I have actual proprietary test data from a set of servovalves indicating that the flow angle varies in a more or less linear fashion from about 40° to about 80°. I have used both the exponential and linear variations in several modeling efforts, and there is a measurable difference — but, arguably, at times not very significant. My personal suspicion is that the flow force variations are more complex than is currently understood by various modeling experts.
The variation in flow angle is likely to be a complex function of the spool geometry and entrance and exit geometries of the fixed body parts. This has been attested to by certain inventors that have succeeded in creating geometric patterns that can nullify the flow force.
Simulating flow angle
I chose to use a variation on the Merritt model (that the flow angle variation is exponential) in a computer simulation. However, the simulation does not rely on a knowledge of the valve’s internal clearances. It uses exponential angle variation, and the range is from 450° to 700°. According to Merritt, the spool movement required to reach the ultimate 69°angle is a function of the spool-to-bore clearance. The larger the radial clearance, the farther the spool land must open before reaching the 69° terminal angle.
My approach in the computer program is that the user enters a percent of spool travel needed to reach the programmed terminal angle of 70°. Thus, the user needs to have no specific knowledge of the clearances.
To investigate the randomness of tolerance buildup, the safe method is to make simulations with a range of values for such probabilistic parameters as spool-to-bore clearance. In the end, the flow force and how it varies does affect the performance of the valve — that is, the ability to maintain constant load flow. Furthermore, it is unlikely that our models of flow force are anything more precise than reasonable approximations to reality. If the valve exists as hardware, the prudent modeler will always adjust the model to the actual test results. If not, we use the best theories at hand.
However, that does not mean the models are without value. It has been my experience that inclusion of flow forces with reasonable variations will suffice to design and build a prototype valve close to final dimensions. After testing, some design changes are likely, but convergence on a final design can be made much more quickly for having conducted the simulation than if all the “what ifs” are done in the laboratory. Spring characteristics and spool diameter are two engineering parameters that are very good candidates for model analysis.
Unloader valve testing
Figure 1 serves as a starting point for explaining the test method used by manufacturers and users of these valves and for the development of the math model. The basic test parameters are the 4-way spool position, or spool shift (KVPL in Figure 1), and the load pressure. With the bias spring pre-compression adjusted to the desired value, the procedure involves setting the pump output flow, setting the 4-way spool at a starting position, then changing the variable load pressure while measuring flows through the compensator spool metering land, to the load, and from the pump and the differential pressure across the 4-way powered land, the compensator inlet pressure, and the outlet pressure).
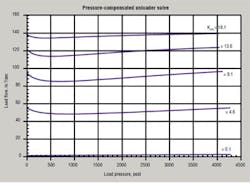
After the load pressure has been adjusted through its full range, it is reduced, and the 4-way spool is shifted to a new position and held there while the load pressure is again adjusted trough the desired range. The result is a set of graphs that display how well the load pressure is maintained at a constant value as the load pressure is varied. The simulated results of this test are shown in Figure 3.
Basic Electronics for Hydraulic Motion Control
This is where your basic electronic training starts. This book, unlike many others, is written specifically by a practitioner of the hydraulic art for engineers and technicians working in hydraulics, but it teaches you all you ever needed to know about electronics. Your personal understanding of hydraulics is your ticket to learning electronics with this book, which is filled with analogies to simplify the understanding of concepts.
Everything you wondered about and need is here: transducers, interfaces, signal conditioners, pulse width modulation, controllers, amplifiers, transistors, analog electronics, digital electronics, and much, much more. It is a must read and reference for anyone interested in certification in the exploding technology of electrohydraulics.
It uses your knowledge of fluid power to help you learn and understand electronics. Order your copy now and get the book that makes electronics fun and easy to learn.
Authored by Jack L. Johnson, P.E., edited by Hydraulics & Pneumatics, and published by Penton Media, the hard cover textbook (ISBN 0-932905-07-2) contains 438 pages and sells for $39.95, plus shipping and sales tax. To order, visit our Bookstore. Print the PDF order form, fill it out, and mail, fax, or e-mail it to us.