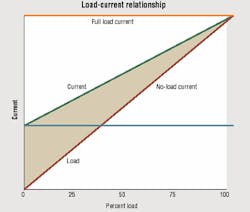
The shaded area represents the margin for error if no-load current is not measured.
Contrary to popular opinion, bigger is not always better. A case in point is the electric motor. Pump users tend to want a little extra power, just in case. Thats why automobile manufacturers can still sell cars with 300 hp engines, when the speed limit may be less than 70 miles per hour. But, like those gas-guzzlers, oversized electric motors cost more to run — sometimes a lot more. In addition to wasting energy, they often trigger expensive demand charges on utility bills due to low power factor.
Fortunately, its easy to determine how much power a pump load requires — without expensive equipment or engineering expertise. In collecting the data, just make sure the pump motor is operating at peak continuous load.
What load is on the pump motor?
The illustration on the next page shows the essentially linear relationship between percent load and current from no-load current to nameplate current of a pump motor. Notice, though, that zero load does not equal zero current. Assuming it does equal zero will cause mistakes in determining the required power, with the error inversely proportional to the load. That means that the biggest errors will occur when evaluating motors most in need of being matched to the pump load.
Although the percent load a motor carries could be determined from a graph, it is easy (and more accurate) to calculate the actual load using the following equation:
hpr = hpn (1 - FLAa - FLAnl)
where hpr is required power in hp,
hpnis nameplate power in hp,
FLAa is actual current draw in amperes
and FLAnl is current drawn at no load in amperes.
| Letter Designation | kV x a/hp |
| A | 0.0 to 3.15 |
| B | 3.15 to 3.55 |
| C | 3.55 to 4.0 |
| D | 4.0 to 4.5 |
| E | 4.5 to 5.0 |
| F | 5.0 to 5.6 |
| G | 5.6 to 6.3 |
| H | 6.3 to 7.1 |
| J | 7.1 to 8.0 |
| K | 8.0 to 9.0 |
| L | 9.0 to 10.0 |
| M | 11.2 to 12.5 |
| N | 12.5 to 14.0 |
| P | 14.0 to 16.0 |
| R | 16.0 to 18.0 |
| S | 18.0 to 0.0 |
| T | 22.0 to 22.4 |
| U | 22.4 to higher |
To obtain good input data, run the motor uncoupled at no load and measure the current with a clamp-on ammeter. Dont take any shortcuts here. The no-load current will be higher if the motor is coupled than if uncoupled. Even though the driven equipment (pump) might not be doing any work, some power is required to overcome friction in driving it. To avoid errors, use the uncoupled current .
Next, document the nameplate current and the current at the motors actual load. Because an undersized motor presents other problems, the safest method is to measure the current over the entire cycle of operation. If the load is seasonal, record the current during peak load.
A realistic example
Its not hard to find oversized motors in industry. In one case, a plant had a 40-hp motor driving a hydraulic pump for a molding machine. The motor had a nameplate current rating of 50 A and drew 15 A when operating uncoupled — slightly more than one-third of fullload current. When driving its peak load, it drew 37 A. A quick calculation reveals that the actual peak load was just over 25 hp:
hpr = 40[1-(50-37) / (50-15)]
hpr = 25.1 hp
Substituting the 40-hp motor with a 25-hp one decreased fullload current from 37 A to 30 A. The plant had been paying for, and wasting, a lot of electricity.
How much does the safety margin cost?
Is it better to oversize the motor for a little extra power, just in case? Consider this. Utilities often impose demand charges for poor power factor when a motor is seriously under-utilized. They also may subject cyclical power users to demand charges based on peak usage. The way it usually works is that one episode of high usage raises the kW/hr cost of electricity for the entire billing period. This imposes severe penalties for starting large motors across the line. Identifying oversized motors can help many users reduce peak demand charges.
The letter designations for locked rotor kV A / hp as measured at full voltage and rated frequency are shown in the table. To calculate the range of inrush current (locked rotor amps) for a motor, determine its NEMA code letter from the nameplate and solve the following equation for the corresponding kV A / hp values shown in the table:
C LR = CL x hp x 1000 / (1.732 x V) where CLR is locked-rotor current,
CL is kV A/hp from the table,
hp is the motor horsepower, and
V is the voltage.
For a 40-hp motor with letter G designation (5.6 - 6.3 kV A/hp), the locked-rotor current will be 281 to 316 A:
CLR= 5.6 x 40 x 1000 / (1.732 x 460)
= 281 A
CLR= 6.3 x 40 x 1000 / (1.732 x 460)
= 316 A
Based on the previous example, substituting a 25-hp replacement motor (code letter F) for the oversized 40-hp model not only decreases full-load current from 37 to 30 A, but also drops starting current from 299 to 188 A. These factors help reduce wear and tear on motor starters, contacts, and other parts from unnecessarily high inrush currents. The substitution also improves power factor appreciably.
Power factor and efficiency
Power factor goes hand-in-hand with efficiency, so its no surprise that the power factor for the 3- phase, 40 hp motor in our example is 0.76 when driving the 25.1 hp load:
E = Wout / Win
= 746 x hp / (1.732 x V x A x PF
= 746 x 25.1 / (1.732 x 460 x 37 x 0.76)
= 18,725 / 22,404
= 0.836, or 83.6% efficiency.
By comparison, the replacement 25-hp motor operates very efficiencly:
E = 746 x hp / (1.732 x V x a PF)
= 746 x 25.1 / (1.732 x 460 x 30 x 0.84)
= 18,725 / 20,077
= 0.933, or 93.3% efficiency.
The power factor can be measured directly with a power factor meter. If a power factor meter is not available, but a watt meter is, the following equation can be used to calculate the power factor of a 3-phase motor:
PF = Win / V-ain
= 22404 / (1.732 x 460 x 37)
= 22404 / 29479
= 0.76
The original 40-hp motor was operating at only about 84% efficiency — nearly 10% below the 93% efficiency of the correctly sized premium-efficient replacement motor. According to the MotorMasterPlus software available from the U.S. Department of Energy ( DOE), if the motor operated 8760 hr/ yr (thats 24/7), the original motor would have used more than 196,000 kW•hr per year, versus 176,000 kW•hr/yr for the 25-hp premium efficient model. At $0.07/kWh, thats a savings of more $ 1400/ yr, every year. Where do you want to spend your money?